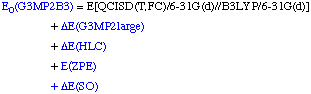G3(MP2)B3 Theory
G3(MP2)B3 is an adaption of G3(MP2) theory based on geometries and zero point vibrational
energies calculated at the Becke3LYP/6-31G(d) level of theory. The G3(MP2)B3 energy at
0 degrees Kelvin E0(G3MP2B3) is defined as:
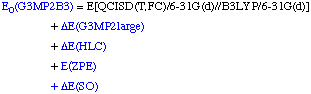
The definition of the components being:
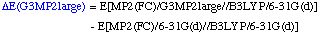


The necessary energies can be calculated most efficiently in the following sequence:
- Optimization and frequency calculation at the Becke3LYP/6-31G(d) level of theory
- QCISD(T,FC)/6-31G(d)//B3LYP/6-31G(d) single point
- MP2(FC)/G3MP2large//B3LYP/6-31G(d) single point
Comments:
- Open shell systems are treated using unrestricted Kohn-Sham orbitals (UB3LYP).
- The higher level correction (HLC) is supposed to compensate remaining deficiencies
of the method. In contrast to G2 theory, separate parameters A and B have been optimized
for atoms and molecules to give the smallest average absolute deviation from experiment.
- Spin orbit correction terms E(SO) (mainly of experimental origin) are added only for atoms.
- The G3MP2large basis set is almost identical to the G3large basis used in G3 except that
core polarization functions are not included.
- The mean absolute devitiation for the extended G2 neutral set (148 reaction energies)
is 1.13 kcal/mol.
Literature:
- L. A. Curtiss, K. Raghavachari, P. C. Redfern, V. Rassolov, J. A. Pople,
"Gaussian-3 (G3) theory for molecules containing first and second-row atoms"
J. Chem. Phys. 1998, 109, 7764 - 7776.
- L. A. Curtiss, K. Raghavachari,
"G2 Theory"
The Encyclopedia of Computational Chemistry, P. v. R. Schleyer (editor-in-chief),
John Wiley & Sons Ltd, Athens, USA, 1998, 2, 1104 - 1114.
- A. G. Baboul, L. A. Curtiss, P. C. Redfern, K. Raghavachari,
"Gaussian-3 theory using density functional geometries and zero-point energies"
J. Chem. Phys. 1999, 110, 7650 - 7657.
last changes: 01.04.2008, AS
questions & comments to: axel.schulz@uni-rostock.de