Electron
Correlation
Within the HF method, the total, antisymmetric, wave function is approximated by a single Slater determinant (single-determinant approximation). At this point, it must be said that exact wave functions cannot generally be expressed as single determinants. The primary deficiency of the HF procedure is the inadequate description of the correlation between electrons. A priori, the single-determinant approximation takes no account of e.g. correlation between electrons with opposite spin, thus leading to a total electronic energy which does not equal the exact solution of the non-relativistic Schrödinger equation, within the Born Oppenheimer approximation. The calculated HF limit is always above the exact energy. The difference
![]()
is called correlation energy, which was introduced by Löwdin.
Using the Roothaan procedure, which linearizes the HF problem, the so-called HF limit can be obtained, if an infinite number of basis functions is used (See Figure).
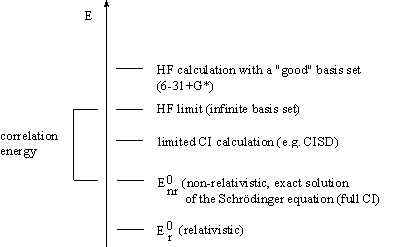
All methods which improve the HF method, deliver, besides the correlation energy, the correlation function:
![]()
A certain amount of electron correlation is already considered within the HF theory, as can be seen in the electron exchange term (correlation between parallel spin electrons) of the expectation value. This prevents two parallel-spin electrons being found at the same point in space. This kind of correlation is often referred to as Fermi correlation. The major deficiency is the neglect of the correlation between the spatial position of electrons with opposite spin due to their Coulomb repulsion. This is called Coulomb correlation.
Within HF theory, the interaction of one electron with the remaining (n-1) electrons is approximated by the interaction of the electron with the average field generated by the (n-1) electrons. This field is static and neglects the influence of the motion of the one electron on the motion of the (n-1) electrons and vice versa, that is, the motion of electrons is correlated.
The term correlation stems from mathematical statistics and means that two distribution functions, f(x) and g(y), for two variables, x and y, are not independent of each other; they are correlated; that is, we cannot write for a joint distribution function:
![]()
Considering two electrons, a and b, it can be said that the probability of finding electron a at a certain position in space, depends on the position of electron b and vice versa. In the statistical sense, for independent electrons:
![]()
where r(1,2) represents the joint density and the factor (n-1)/n accounts for the indistinguisability of electrons. r(1,2)dx1dx2 [x = (r,s)] determines the probability of two electrons being found simultaneously in the elements dx1 and dx2. The product of the density functions r(1)r(2) does not adequately describe the "real" situation. For small distances, the pair density, r(1,2) , is too large and similarly, too small for large distances. That is, the electrons "avoid" each other. This is what is meant by electron correlation. If the electrons were not correlated, each electron could be described by a "charge cloud" (density) and the only interaction between the two electrons would be the Coulomb repulsion between these densities. However, the actual electron repulsion is less than it should be according to this model, because electrons avoid each other. That is, they never come as close together as statistically independent particles would do. This decreased repulsion, due to the correlation of the electron motion, is partly responsible for the correlation energy.
For the correlation of the electrons in space, there are essentially two reasons. One is that an antisymmetric wave function (See Pauliís principle) must be used for electrons (fermions) and the associated correlation is the Fermi correlation. The other is the Coulomb correlation, corresponding to the Coulomb repulsion of the electrons. Besides these two effects, there is a correlation related to the overall symmetry, or total spin of the considered state.
The electron density r(1) at the position r1 can be described as a sum of a density with a and b spin:
![]()
ra(1) [ rb(1)] corresponds to the probability density of finding an electron with a [b] spin at the position r1. Analogously, rab(1,2) describes the probability density of finding an electron with a spin at the position r1 and simultaneously, a second electron with b spin at the position r2 . The product, r(1)*r(2), can be written as:
![]()
Recall that antisymmetry demands, see Pauliís principle:
![]()
The probability of finding two a (or b) spin electrons at the same position (r1=r2 ) vanishes. The displacement between two electrons cannot become infinitely small. That is to say, in the distribution function of each electron, there exists a "hole", the Fermi hole, in which, at a certain time, the electron cannot "stay" (Fermi correlation).
In contrast to the pair density, raa(1,2), the pair densities, rab(1,2) and rab(1,2) , do not vanish for the limiting case; however, the probability density does drop to a minimum value at this point. This is referred to as a Coulomb hole. Hence, close to the point r1 = r2, the rab(1,2) density is smaller than the product ra(1)*rb(2) (Coulomb correlation). The neglect of the (Coulomb) correlation leads to an artificial decrease of the electron-electron distance and therefore, to an electronic energy which lies above the exact energy eigenvalue and to shorter calculated bond lengths. The absolute value of the correlation energy in a quantum mechanical system is often not very large, usually of the order of about 1% of the total energy. For example, the electron correlation of the H2O molecule is roughly 0.5% of the total energy. However, chemical bond energies and spectroscopic energy differences are also very small compared to the total energy of a molecular system. In this case, the correlation energy cannot be neglected. Typically, bond energies lie in the range of one percent of the total energy. An 1% error in the determination of the total energy could lead, to as much as an, 100% error in the calculation of the bond energy.
Having only a small influence on the calculated structure of molecules in most cases, the correlation energy is very important when investigating the thermodynamics of reaction mechanisms, in which bond dissociation and bond formation occur, and in particular, the energy of transition states is required.
Qualitatively wrong results will be derived from HF calculations in which the dissociation of molecules is considered, in cases were the dissociation does not lead to closed-shell fragments. (The closed shell HF determinant often does not dissociate correctly when nuclei are moved to infinite separation e.g. dissociation of H2). This failure lies in the nature of the single-determinant approximation, which must fail for any degenerate state.
Often, electron correlation is divided into dynamic correlation and non-dynamic correlation which represent two different approaches to the same problem. Dynamic correlation, which is the larger of the two, is responsible for obtaining the "correct" distance between electrons, if in the wave function, higher excitations are included (See Configuration Interaction, CI Method). By this extension of orbital space, additional interactions (fields) are introduced. If all excitations are taken into account, then each possible field interacts proportionately with the electron corresponding to the above-discussed correlated motion. Non-dynamic correlation means that using the single Slater determinant of the HF approximation as a reference function is no longer sufficient to generate a CI wave function as a linear combination of the HF determinant and a limited number of excited states (built from the HF orbitals). This is not a dynamic effect and has its origin in the correlated motion of electrons. To avoid this effect, a limited CI calculation with several reference determinants should be performed (MRCI).
The explicit consideration of correlation energy can be often avoided
by using isodesmic equations. In an isodesmic reaction, the total number
of each type of bond on the reactant side and product side remains constant.
In such a reaction, where the type and the number of bond-electron pairs
remains equal, it can be assumed, that the error on both the educt and
product side, is quite similar and cancellation of the error will lead
to reasonably good reaction energies. Moreover, comparing very similar
systems allows maximum advantage to be taken of this cancellation error.
post-HF methods - general considerations
DFT
Limitations of post HF methods
last changes: 01.04.2008, AS questions & comments to: axel.schulz@uni-rostock.de