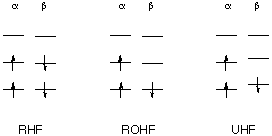In Hartree-Fock calculations on closed shell systems (even number of electrons, all electrons paired) the electrons of opposite spin (spin-up, alpha vs. spin-down, beta) occupy the same spatial orbitals. The restriction of using the same spatial orbitals is usually reflected in the acronym RHF (=restricted Hartree-Fock) for these types of calculations. Once the numbers of alpha and beta spin electrons become different, however, this is not necessarily the best solution. If the restriction of identical spatial orbitals is retained in this situation, the method is called ROHF (Restricted Open Shell Hartree-Fock). If alpha and beta spin electrons are allowed to occupy different spatial orbitals the method is referred to as UHF (Unrestricted Hartree-Fock) method. The following scheme illustrates the different methods:

While UHF calculations on open shell systems usually give lower energies and a better description of the unpaired electron density distribution (and thus EPR spectra), the UHF wavefunction is not an eigenfunction of the <S²> operator. In particular for spin-delocalized systems such as allylic or benzylic radicals, the UHF wavefunction can deviate substantially from that for a doublet state. The degree of deviation can be characterized through the difference between the expectation value of the <S²> operator (given after the SCF convergence note in the output file) and the value of S(S+1) for the current spin quantum number of the system. For a doublet state S=0.5 and S(S+1) = 0.750. The allyl radical will be used here to illustrate the situation at the UHF/STO-3G level of theory:

In this particular case, using the structure obtained at the UHF/STO-3G level of theory, the UHF/STO-3G total energy is somewhat lower at -115.054291242au than the ROHF/STO-3G total energy at -115.008930049au (= -119.1 kJ/mol). The expectation value of the <S²> operator is 1.1009 at the UHF/STO-3G and 0.7500 at the ROHF/STO-3G level. This constitutes a severe case of spin contamination of the UHF wavefunction.